
Here we will learn how to convert to and from standard form, including how to adjust numbers to write them in standard form notation.
There are also converting to and from standard form worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
Converting to and from standard form is where we convert an ordinary number to a number written in standard form or scientific notation.
Standard form is a way of writing very large or very small numbers by comparing the powers of ten.
Numbers in standard form are written in this format:
Where a is a number 1\leq\lt10 and n is an integer.
Converting between ordinary numbers and numbers in standard form can help us to compare numbers and interpret answers given in standard form on a calculator.
To do this we need to understand the place value of a number.
E.g.
Let’s look at the number 350000 and place the digits in a place value table:
So 350000 written in standard form is:

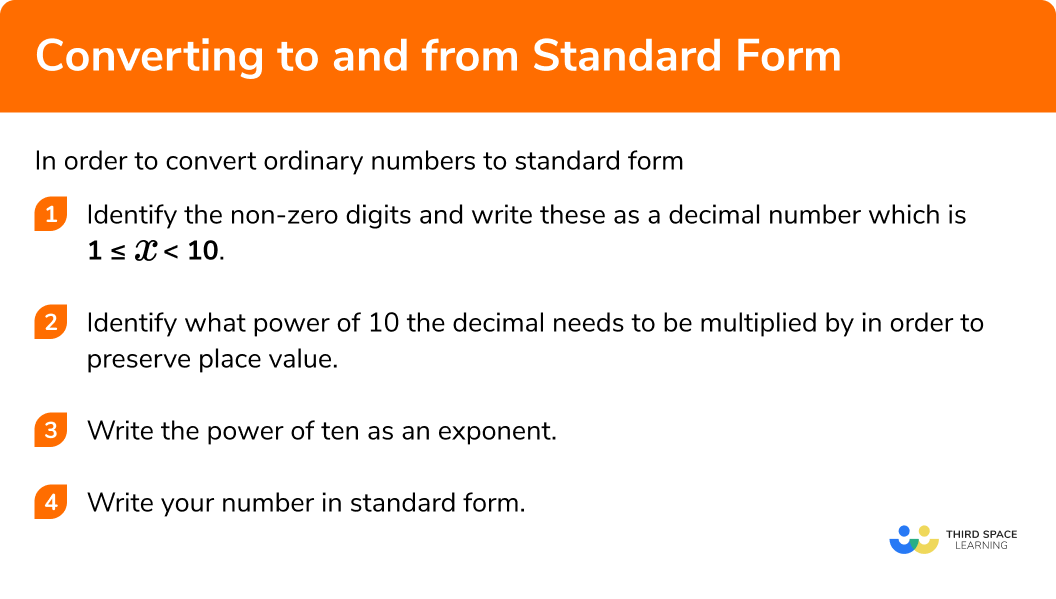
In order to convert ordinary numbers to standard form:

Get your free standard form calculator worksheet of 20+ questions and answers. Includes reasoning and applied questions.


Get your free standard form calculator worksheet of 20+ questions and answers. Includes reasoning and applied questions.
Write this number in standard form:
The number needs to lie between 1\leq\lt10
So the number will begin as 4.8… .
2 You now need to maintain the value of the number by multiplying that decimal by a power of ten.
\[4.8 \times 100000 = 480000\]3 Write that power of ten as an exponent.
\[100000 = 10^<5>\]4 Write your number in standard form.
Write this number in standard form:
The non-zero digits need to be written in decimal notation.
The number needs to lie between 1\leq\lt10
So the number will begin as 5.42… .
You now need to maintain the value of the number by multiplying that decimal by a power of ten.
\[5.42 \times 1000000 = 5420000\]Write that power of ten as an exponent.
\[1000000 = 10^<6>\]Write your number in standard form.
\[5.42\times10^<6>\]Write 0.00081 in standard form.
Identify the non-zero digits and write these as a decimal number which is \pmb<1\leq
This number will begin with 8.1.
Identify what power of ten the decimal needs to be multiplied by in order to preserve place value.
8.1 \div 10000 = 0.00081
Write the power of ten as an exponent.
Write your number in standard form.
Write 0.00718 in standard form.
Identify the non-zero digits and write these as a decimal number which is \pmb<<1\leq
The number will begin with 7.18…
Identify what power of ten the decimal needs to be multiplied by in order to preserve place value.
7.8 \div 1000 = 0.0078
Write the power of ten as an exponent.
Write your number in standard form.
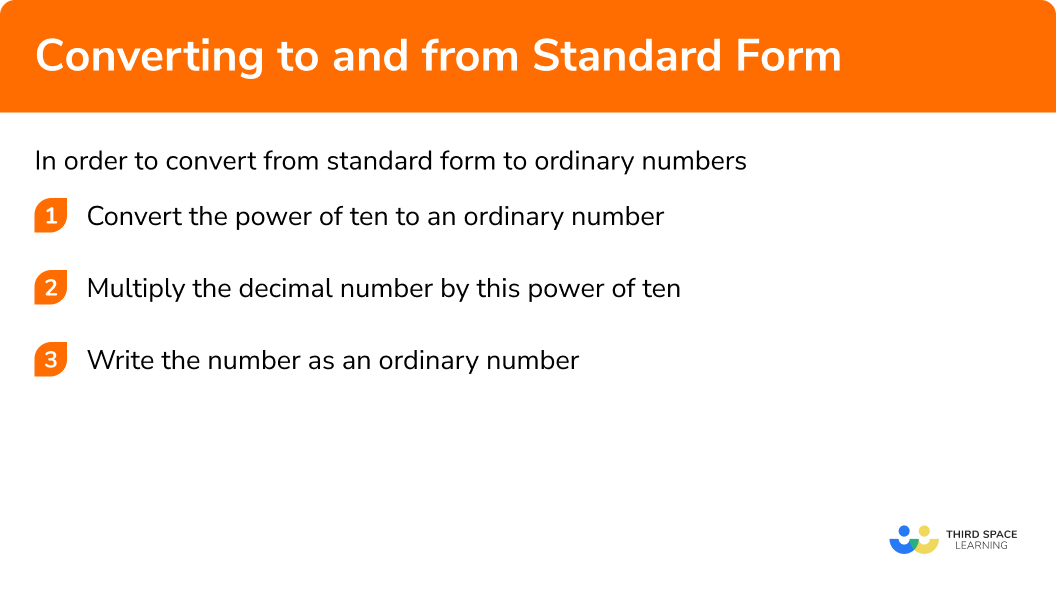
\[7.18\times10^<-3>\]In order to convert from standard form to ordinary numbers:
Write 6.2\times10^4 as an ordinary number.
Write the exponent as a power of ten.
Multiply the decimal number by that power of ten.
\[6.2 \times 10000\]Write your answer as an ordinary number.
\[6.2 \times 10000 = 62000\]Write 1.9\times10^ as an ordinary number.
Write the exponent as a power of ten.
Multiply the decimal number by that power of ten.
\[1.9 \times 0.001\]Write your answer as an ordinary number.
\[1.9 \times 0.001 = 0.0019\]Sometimes we might have a number that looks like it is in standard form however the decimal number is not between 1 and 10 ,
E.g.
36103 or 0.2104 .
In this case we need to adjust the number.
In order to adjust numbers to standard form:
Write 48\times10^5 in standard form.
Identify what the first number needs to be multiplied or divided by so that it lies between \pmb<1\leq
48 needs to be divided by 10 so 48 becomes 4.8 .
Apply the inverse operation to the power of ten.
10^5 needs to be multiplied by 10 which adds one to its power, so it becomes 10^6 .
Write your number in standard form.
Write 0.68\times10^ in standard form.
Identify what the first number needs to be multiplied or divided by so that it lies between \pmb<1\leq
0.68 needs to be multiplied by 10 so it becomes 6.8 .
Apply the inverse operation to the power of ten.
10^4 needs to be divided by 10 which subtracts one from its power, so it becomes 10^3 .
Write your number in standard form.
Write 290\times10^ in standard form.
Identify what the first number needs to be multiplied or divided by so that it lies between \pmb<1\leq
290 needs to be divided by 100 so it becomes 2.9 .
Apply the inverse operation to the power of ten.
10^ needs to be multiplied by 100 which adds two to its power, so it becomes 10^ .
Write your number in standard form.
This error is often made by counting the zeros following the first non zero digit for large numbers or zeros after the decimal point for small numbers, then writing this as the power, rather than considering the place value of the given number.
In a number such as 0.000682 , selecting the ‘2’ to determine the exponent rather than the ‘6’ which has a higher place value.
In standard form, this number would be 6.82 × 10^ .
When checking the standard form of a number, incorrectly adjusting the negative powers due to not applying negative numbers rules correctly.
E.g.
With small numbers, adding one to the power of 10^ will result in 10^ not 10^ .
Converting to and from standard form is part of our series of lessons to support revision on standard form. You may find it helpful to start with the main standard form lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:
1. Write 270000 in standard form